Virtual Labs

1. Which of the following code can't be an MDS code?

(7,3,5)

Explanation

(63,57,7)

Explanation

(255,249,7)

Explanation

(15,8,7)

Explanation

2. The code

(5,2)

over the field

\mathbb{F}_{7}

generated by the generator matrix

G = \begin{bmatrix} 2 & 0 & 1 & 4 & 3 \\ 5 & 6 & 2 & 1 & 0 \end{bmatrix}

is MDS.

a: True Explanation

Explanation

b: False Explanation

Explanation

c: Data Insufficient Explanation

Explanation

3. The elements of

\mathbb{F}_{2^2}

are given in Table 2. Suppose for the

(3,2)

code the message bit stream is

1011

, i.e.,

u_{0}= 10

and

u_{1}= 11

. Then the corresponding message symbols in the field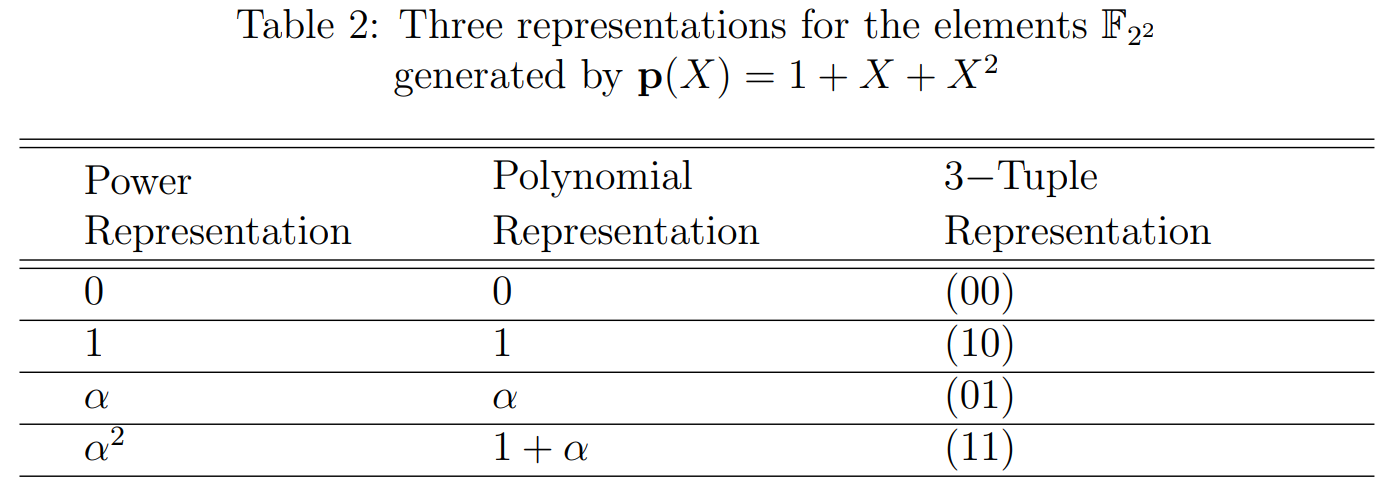

\mathbb{F}_{2^2}

\\

u_{0}= \alpha, u_{1}= \alpha^{2}

Explanation

u_{0}= \alpha, u_{1}= 1

Explanation

u_{0}= 1, u_{1}= \alpha^{2}

Explanation

d: None of the above Explanation

Explanation

4. The generator matrix of the

(3,2,2)

RS code over

\mathbb{F}_{2^{2}}

G = \begin{bmatrix} 1 & 0 & 1 \\ 0 & 1 & 1 \end{bmatrix}

Explanation

G = \begin{bmatrix} 1 & 0 & \alpha^{2} \\ 0 & 1 & \alpha \end{bmatrix}

Explanation

G = \begin{bmatrix} 0 & \alpha & \alpha^{2} \\ 1 & \alpha & \alpha \end{bmatrix}

Explanation

G = \begin{bmatrix} 1 & 1 & 1 \\ 1 & \alpha & \alpha^{2} \end{bmatrix}

Explanation

5. For the

(3,2,2)

RS code over

\mathbb{F}_{2^{2}}

the encoded bit stream of

1011

000101

Explanation

010011

Explanation

010001

Explanation

011001

Explanation

6. Which of the following is evaluation set of

(6,3,4)

RS code over the filed

\mathbb{F}_{2^{3}} = \{ 0, 1, \alpha, \alpha^{2}, \alpha^{3} = 1+\alpha, \alpha^{4} = \alpha+\alpha^{2}, \alpha^{5}=1+\alpha+\alpha^{2}, \alpha^{6}= 1+\alpha^{2} \}

\{ \alpha, \alpha^{3}, \alpha^{4}, \alpha^{5}, \alpha, 1 \}

Explanation

\{ \alpha, \alpha^{3}, \alpha^{4}, \alpha^{5}, \alpha^{6}, 1+\alpha \}

Explanation

\{ \alpha, \alpha^{3}, \alpha^{4}, \alpha^{6}, 1 \}

Explanation

\{ \alpha, \alpha^{3}, \alpha^{4}, \alpha^{5}, \alpha^{6}, 1 \}

Explanation

Reed-Solomon Codes