Fermi Level

The fermi function gives the probability of a state(if it exists) being occupied at equilibrium.

f_o(E) = 1 / [1 + e^{(E - E_f) / k_BT}]

The parameters of the function are, f_o(E) and T.

It also describes the electron density in the conduction band and the hole density in the valence band.

The fermi level is the value of Ef when the fermi function equates to ½, i.e.

f_o(E) = 1/2

States below the fermi level have a low probability of being empty and the states above the fermi level have a low probability of being filled.

The width of the transition depends on temperature. The transition between high probability and low probability states increases with increase in temperature.

Equilibrium Carrier Densities

Equilibrium carrier densities refer to the number of carriers in the conduction and valence band with no externally applied bias.Non-degenerate semiconductors are defined as semiconductors for which the Fermi energy is at least 3kT away from either band edge. It essentailly is a semiconductor whose conduction band level is much higher than the fermi-level and whose valence band level is much lower than the fermi-level. In a non degenerate semiconductor, the probability of the state at the bottom of the conduction band can be approximated to e^{(E_f - E_C) / k_BT}. Therefore, n, the electron density, is proportional to this value. Similarly, the probability of the state at the top of the valence band can be approximated to e^{(E_V + E_f) / k_BT}. Therefore, p, the electron density, is proportional to this value.

n ∝ e^{(E_f - E_C) / k_BT}

p ∝ e^{(E_V - E_f) / k_BT}

Where, n is electron density at the the bottom of the conduction band p is the electron density at the top of the valence band E_f is the fermi level E_C is the fermi energy of the conduction band E_V is the fermi energy of the valence band

Electron Density in the Conduction Band

The number of electrons in a region can be calculated as the product of the number of states in that region and the fermi function(gives probability of those states being occupied).

n_o = ∫f_o(E)D_C(E) dE

f_o(E) = 1/(1+e^{(E - E_f) / k_BT})

D_c = (^(2m*)3/2 / 2π²ℏ³) √(E - E_C)

To calculate the electron density in the conduction band,

n_o = E_Cn_o(E) dE = E_Co(E)D_C(E) dE

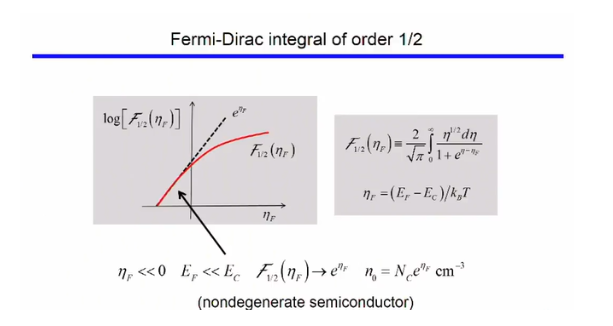
This can be approximated to

n_o = N_C F_1/2(η_F)

Here F_1/2 is the fermi-dirac integral. Where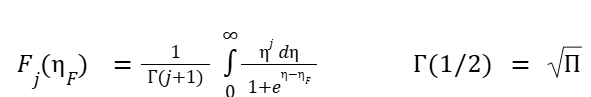

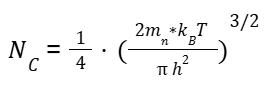For a nondegenerate semiconductor, dF_j dη_F;= F_j+1 Therefore, F_1/2(F) can be approximated to eF for nondegenerate semiconductors. n_o = N_C e^η_F Where, and F = (E_F + E_C) / k_BT

Hole Density in the Valence Band

Similar to electron density, hole density is found to be the product of the number of states in that region and the fermi function(gives probability of those states being occupied).

p_o = N_V e^η_F

Where,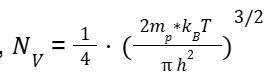 and F = (E_V - E_F) / k_BT

Equilibrium Carrier Density Product

This can be found by multiplying electron and hole concentrations

This expression can be written as

Where n_i is the intrinsic carrier concentration and E_G is the band gap. The intrinsic carrier concentration is not dependent on the fermi level. It also depends exponentially on band gap and temperature.

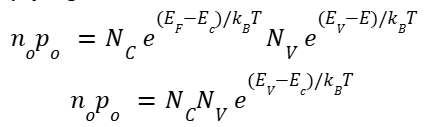
Intrinsic Fermi level

For an n-type semiconductor, the fermi level is closer to the conduction band because the number of electrons in the conduction band is much larger than the number of holes in the valence band. Similarly, the fermi level of a p-type semiconductor is closer to the valence band because the number of holes in the valence band is much larger than the number of electrons in the conduction band. So for an intrinsic semiconductor, where the number of electrons and holes are equal, we can deduce that the fermi level should be right in the middle.

However, there is an additional correction factor. This correction depends on the effective densities of states in the valence and conduction bands.

Doping Density

In a uniformly doped semiconductor, mobile charges(electrons or holes) will be attracted to stationary charges (immobilized ions and dopants) so the net charge is zero.

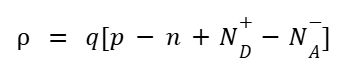
Almost all semiconductors will be nearly neutral in charge but with strong non-uniformities (like the p-n junction), there will be a local charge.

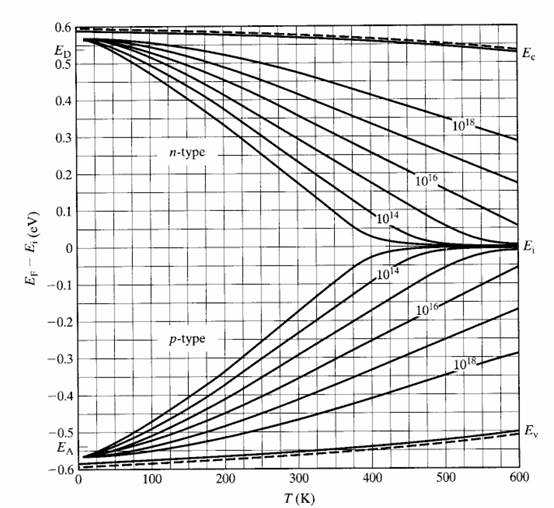
Doping and Temperature

The Intrinsic Region

Intrinsic carriers are created by breaking covanlent bonds and exciting electrons accrossthe bandgap.
Dopants are fully ionized and the net doping density is much larger than intrinsic carrier concentration.

(n_i²/n_o) - n_i+N_D N^{λ _{= 0}}

(n_i²/n_o) - n_i + N_D - N_λ = 0

n_o = k + (k² + n_i²)^1/2

where k = (N_D - N_λ)/2

p_o = n_i²/n_o

At higher temperatures, when semiconductors go intrinsic,the intrinsic carriers overwhelm the dopants. Devices, which rely on selective doping fail at high temperature(500k or above). However, semiconductores with larger bandgaps can operate in higher temperatures.

The Extrinsic Region

Dopants are fully ionized. The majority carrier concentration is simply equal to the dominant doping concentration. The minority carrier concentration equals n_i² divided by the dominant doping concentration.
For an n type doped device,

N_D - N_λ >> n_i

n_o = N_D - N_λ

p_o = n_i²/n_o

Similarly for a p type doped device,

N_D - N_λ >> n_i

p_o = N_D - N_λ

n_o = n_i²/p_o

Freeze Out Region

At very low temperatures (large 1/T), negligible intrinsic electron-hole-pairs (EHPs) exist (ni is very small), and the donor electrons are bound to the donor atoms. This is known as the ionization (or freeze-out) region.
Dopants are partially ionised. The majority charge carrier concentration makes up almost all of the charge carrier concentration.
Although metals can conduct at 0 Kelvin, semicondonctures cannot.
However, heavily doped semiconductors are an exception. At large dopant concentrations, impurity ion distribution causes significant fluctuations in the local electrostatic potential, which gives rise to a spacial variation in th elocal density of states distribution. When averaged over the entire lattice, the conduction band and valence band essentially merge.