Procedure –

Experimental Setup

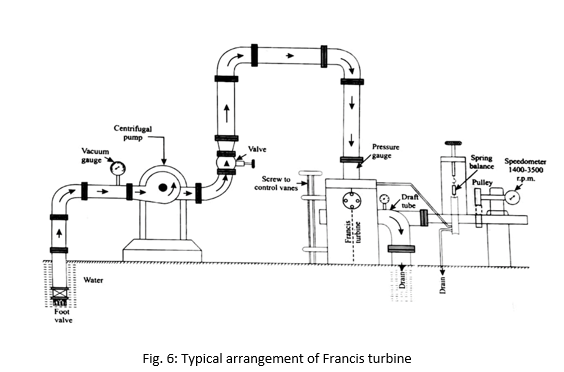
1. A Francis turbine with an arrangement for adjusting the guide vane positions (hand wheel with suitable link mechanisms)
   
   
2. Supply pump unit.
   
   
3. Flow measurement unit (viz. venturi meter with manometer)
   
   
4. Tachometer.
   
   
5. Pressure gauges at the inlet and outlet of turbine.
   
   
6. Rope brake dynamometer with spring balance connected to the turbine shaft.
   
   

Formulae

1. V₁=Q/(π/4) d₁²
   
   Where V₁ is absolute velocity of water entering
   
   V₂ is absolute velocity of water leaving
   
   Q= discharge
   
   d₁= Dia. of Inlet pipe
   
   d₂= Dia. of outlet pipe
   
   
2. H= (P₁/w-P₂/w)+ (V₁²- V₂²/2g)+ (Z₁- Z₂ )
   Where, Z₁ = Distance of inlet pressure gauge from turbine axis
   
   Z₂ =Distance of inlet pressure gauge from turbine axis w = Specific weight of water
   
   
3. Input power: P_i=(w×Q×H)/75
   
   
4. Output Power: P_o=((W-S)×π×D×N)/4500
   
   Where, D = Mean Diameter of the break drum
   
   N = Speed of motor in RPM
   
   
5. η=P_o/P_i ×100
   
   

Procedure –

1. Note the inlet and outlet pipe diameters and measure the brake drum diameter and Z₁ and Z₂ i.e. the distances of inlet and outlet pressures gauge tapings from the centerline of the turbine.
   
   
2. Start the supply pump, keeping the guide vanes completely closed
   
   
3. Open the guide vanes partially (e.g. 1⁄4 or 1⁄2 of total opening),
   
   
4. Measure the discharge Q
   
   
5. Note the readings of pressure gauges
   
   
6. Note the readings of W (load on hanger) and spring balance S and shaft speed N
   
   
7. Vary speed of turbine by varying the load i.e. W and S on the brake drum and take six to seven readings in the allowable range of speed
   
   
8. Change the guide vane opening and repeat steps 4 to 7
   
   

Observations -

1. Mean Diameter of the break drum, D(D_d + D_r) = Where D_d = diameter of the drum, Dr = diameter of rope
   
2. Dia. of Inlet pipe, d₁ = 65 mm
   
3. ia. of outlet pipe, d₂ = 38.45 mm
   
4. Distance of inlet pressure gauge from turbine axis Z₁ =
   
5. Distance of inlet pressure gauge from turbine axis Z₂ =
   
6. Venturi meter constant, k = 35.7 Q=k √h
   
   
   

Calculations

1. V₁=Q/((π/4) 〖d₁〗² ) V₂=Q/((π/4) 〖d₂〗² )
   
2. H= (P₁/w-P₂/w)+ (〖V₁〗²- 〖V₂〗²/2g)+ (Z₁- Z₂ )
   
3. Input power: P_i=(w×Q×H)/75
   
4. Output Power: P_o=((W-S)×π×D×N)/4500
   
5. η=P_o/P_i ×100
   
6. Plot the following curves and determine the efficiency of the Kaplan turbine
   a. Efficiency Vs. Speed
   b. Efficiency Vs. Power Output