Beam Test

Objective:

To study the modes of vibration of simply supported beams under flexure.

Apparatus used:

Simply supported beam

Specimen 1

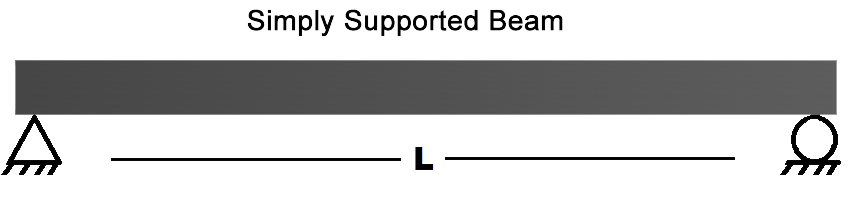
Enter the length of beam, L (in meters) :

*

Enter the breadth of beam, B (in meters) :

*

Enter the depth of beam, D (in meters) :

*

Or

Enter the area of beam, A (in square meters) :

*

Enter the moment of inertia of beam, I (in meters) :

*

Note: Here, moment of inertia is a property of shape that is used to predict deflection, bending and stress in beams.

Step 1 a) Click on add button to bring blocks in the lab. b) Click on add button to bring beam in the lab. c) Click on blocks to arrange them in right position. d) Click on beam to put it on the blocks.

Specimen 1

Enter the density of beam (in kg/m³) :

*

Enter the Young's modulus of elasticity E, of beam (in N/m²) :

*

Select the value of n:

*

Note: Young modulus, is a mechanical property that measures the stiffness of a solid material. It defines the relationship between stress (force per unit area) and strain (proportional deformation) in a material in the linear elasticity regime of a uniaxial deformation.

Specimen 1

Frequency,f_n (in Hz) :

Repeat from step 2 for other two specimen.

Specimen 2

Note: Enter different values of length for different no. of specimen

Enter the length of beam, L (in meters) :

*

Enter the breadth of beam, B (in meters) :

*

Enter the depth of beam, D (in meters) :

*

Or

Enter the area of beam, A (in square meters) :

*

Enter the moment of inertia of beam, I (in meters) :

*

Note: Here, moment of inertia is a property of shape that is used to predict deflection, bending and stress in beam.

Step 2 a) Click on add button to bring blocks in the lab. b) Click on add button to bring beam in the lab. c) Click on blocks to arrange them in right position. d) Click on beam to put it on the blocks.

Specimen 2

Note: Enter different values of Young's modules of elasticity (E) for different no. of specimen

Enter the density of beam (in kg/m³) :

*

Enter the Young's modulus of elasticity E, of beam (in N/m²) :

*

Select the value of n:

*

Note: Young modulus, is a mechanical property that measures the stiffness of a solid material. It defines the relationship between stress (force per unit area) and strain (proportional deformation) in a material in the linear elasticity regime of a uniaxial deformation.

Specimen 2

Frequency,f_n (in Hz) :

Repeat the whole experiment for last one specimen.

Specimen 3

Note: Enter different values of length for different no. of specimen

Enter the length of beam, L (in meters) :

*

Enter the breadth of beam, B (in meters) :

*

Enter the depth of beam, D (in meters) :

*

Or

Enter the area of beam, A (in square meters) :

*

Enter the moment of inertia of beam, I (in meters) :

*

Note: Here, moment of inertia is a property of shape that is used to predict deflection, bending and stress in beam.

Step 3 a) Click on add button to bring blocks in the lab. b) Click on add button to bring beam in the lab. c) Click on blocks to arrange them in right position. d) Click on beam to put it on the blocks.

Specimen 3

Note: Enter different values of Young's modules of elasticity (E) for different no. of specimen

Enter the density of beam (in kg/m³) :

*

Enter the Young's modulus of elasticity E, of beam (in N/m²) :

*

Select the value of n:

*

Note: Young modulus, is a mechanical property that measures the stiffness of a solid material. It defines the relationship between stress (force per unit area) and strain (proportional deformation) in a material in the linear elasticity regime of a uniaxial deformation.

Specimen 3

Frequency,f_n (in Hz) :

Repeat the whole experiment for last one specimen.

Plot graph between Length & Calculated Frequency

Length :	L1 :	L2 :	L3 :
Frequency :	F1 :	F2 :	F3 :

Plot graph between Elasticity & Calculated Frequency

Elasticity :	E1 :	E2 :	E3 :
Frequency :	F1 :	F2 :	F3 :

Thank You for performing experiment, to repeat experiment click Restart button.

Modes of Vibration of Simply Supported Beam Under Flexure